
The same method may be used to find arc length – all you need to remember is the formula for a circle's circumference. So, what's the area for the sector of a circle:įrom the proportion, we can easily find the final sector area formula:
Then, we want to calculate the area of a part of a circle, expressed by the central angle.įor angles of 2π (full circle), the area is equal to πr²: 2 It is used extensively in architecture when calculating the arc necessary to span a certain height and distance and also in optics where it is used to find the depth of a spherical mirror.
Definition of chord geometry full#
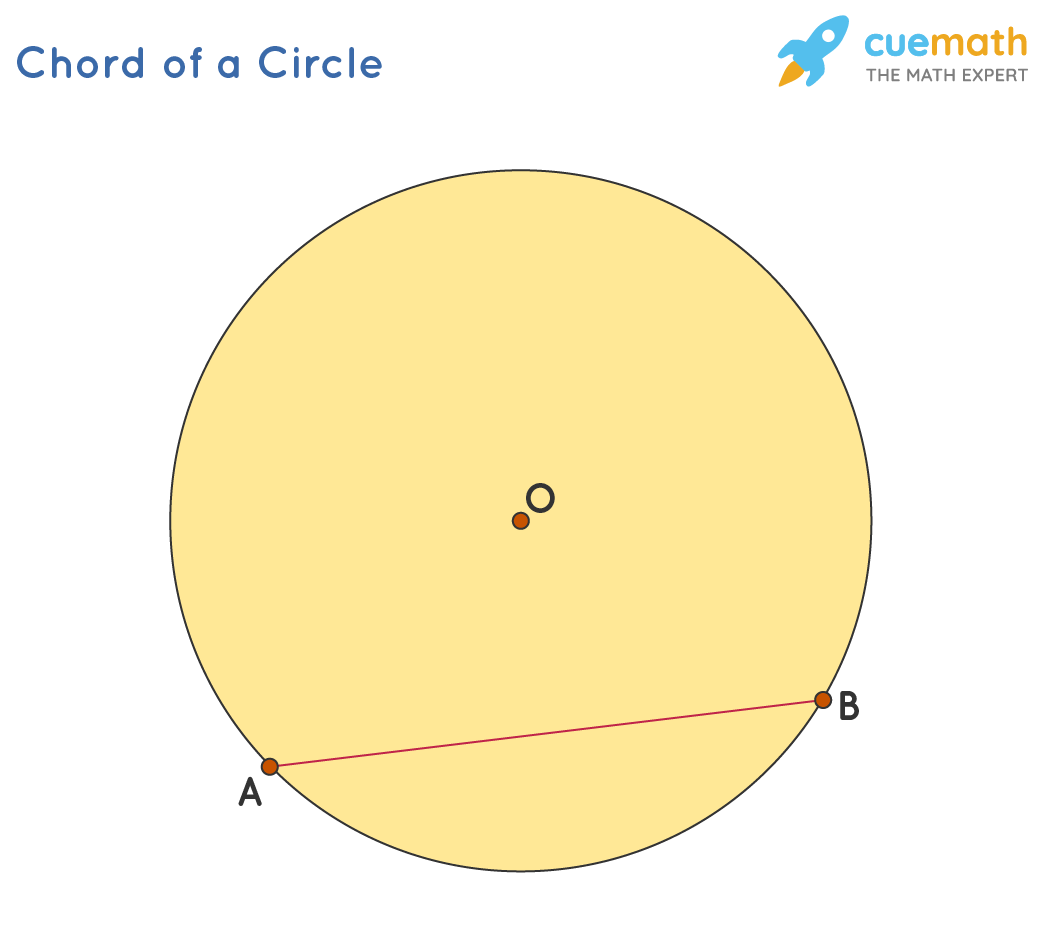
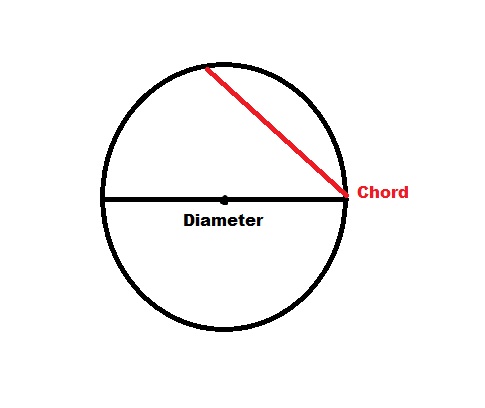
The full angle is 2π in radians, or 360° in degrees, the latter of which is the more common angle unit. In geometry, the sagitta (sometimes abbreviated as sag 1) of a circular arc is the distance from the midpoint of the arc to the midpoint of its chord. The area of a circle is calculated as A = πr². All you need to remember is the circle area formula (and we bet you do!): The intersection of the diameter and the chord at 90 degrees can be very close to the centre and so the two lengths coming from the point of intersection to the radius are assumed to be equal, but they aren’t.The formula for sector area is simple – multiply the central angle by the radius squared, and divide by 2:īut where does it come from? You can find it by using proportions. Incorrect assumption of isosceles triangles.This also includes the inverse trigonometric functions. The incorrect trigonometric function is used and so the side or angle being calculated is incorrect. The missing side is calculated by incorrectly adding the square of the hypotenuse and a shorter side, or subtracting the square of the shorter sides. The only case of this is when both angles are 90^o. Opposite angles are the same for a cyclic quadrilateralĪs angles in the same segment are equal, the opposing angles in a quadrilateral are assumed to be equal.Angle at the centre is supplementary to opposing angleĪs the shape is a quadrilateral, the angle at the centre is assumed to be supplementary and add to 180^o.The angle ABC = 56^o as it is in the alternate segment to the angle CAE. Line Segment - It is the shortest distance between any two. The chord of a circle is a line that joins any two points on the circumference of the circle. Point - It is an exact position and location on a plane surface. Here, angle ABC is incorrectly calculated as 180 - 56 = 124^o. Chord of a Circle: Definition and Formula - Edureify-Blog. The angle is taken from 180^o which is a confusion with opposite angles in a cyclic quadrilateral. Opposite angles in a cyclic quadrilateral.Top tip: Use arrows to visualise which way the alternate segment angle appears: The chord BC is assumed to be parallel to the tangent and so the angle ABC is equal to the angle at the tangent. Parallel lines (alternate segment theorem).The angle at the circumference is assumed to be 90^o when the associated chord does not intersect the centre of the circle and so the diagram does not show a semicircle. They should total 90^o as the angle in a semicircle is 90^o. The angles that are either end of the diameter total 180^o as if the triangle were a cyclic quadrilateral. Look out for isosceles triangles and the angles in the same segment. Make sure that you know when two angles are equal. The angle at the centre is always larger than the angle at the circumference (this isn’t so obvious when the angle at the circumference is in the opposite segment). Make sure you know the other angle facts including:īy remembering the angle at the centre theorem incorrectly, the student will double the angle at the centre, or half the angle at the circumference. Below are some of the common misconceptions for all of the circle theorems:


 0 kommentar(er)
0 kommentar(er)
